PEDAL TRIANGLE

By Dario Gonzalez Martinez

As usual, we should start our mathematic discussion with a definition to ensure that we can understand the concepts involved here. In this manner, we will define what a Pedal Triangle is.

PEDAL TRIANGLE

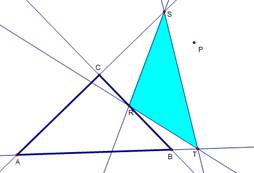
Consider a triangle ABC and a point P on the plane, which could be located anywhere. Construct lines hat pass through P and are perpendiculars to each triangle’s side (or its extensions). Let R, S and T be the intersections between triangle’s sides and each of their perpendicular lines before mentioned. The triangle defined by those three points is the Pedal Triangle of triangle ABC, and the point P is called Pedal Point. Figure 1 shows examples of pedal triangles for different locations of P.

Figure 1(a)	Figure 1(b)

Let’s see what happens with the pedal triangle if the pedal point is located in some special positions. However, if you want to explore in GSP first, you can click here.

PEDAL POINT ON TRIANGLE’S CENTERS

I. Pedal point as Incenter

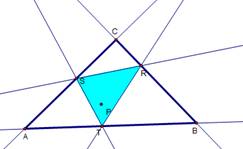
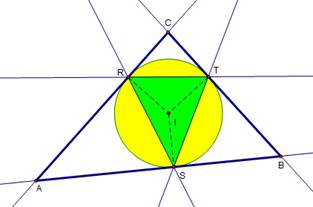
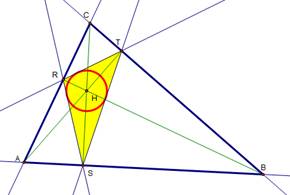
Consider a triangle ABC in which is drawn its incenter and its incircle. We will choose as pedal point the incenter of the triangle ABC. Figure 2 below shows this idea:

Figure 2

The incenter I is the pedal point chosen, so the perpendicular lines to each triangle’ sides are IR, IT and IS, and RST is the pedal triangle. Since the pedal point is the incenter of triangle ABC, it is equidistant from each vertex of triangle RST, which means that the yellow circle (incircle of DABC) is indeed the circumcircle of DRST by definition. Thus the Incenter of triangle ABC is the Circumcenter of pedal triangle RST.

II. Pedal point as Circumcenter

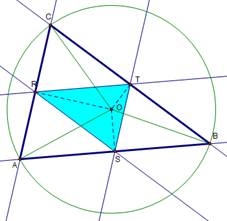
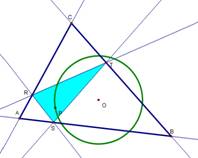
This time, consider a triangle ABC in which is drawn its circumcenter and its circumcircle. We will choose as pedal point the circumcenter of the triangle ABC. Figure 3 below shows this idea:

Figure 3

The figure 3 above suggests that the pedal triangle’s sides are parallel to the triangle ABC’s sides. This is easy to show, we just need to look carefully at the pedal triangle’s vertices R, S and T because they are the midpoints of triangle ABC’s sides. Consider triangle AOB, this triangle is isosceles because OA = OB since they are radius of the circumcircle with center O. So, given that OS is perpendicular to AB by definition, it must be the altitude of triangle AOB from the base AB, so S is midpoint of segment AB. We can elaborate similar arguments to show that R and T are also midpoints of AC and BC, respectively.

Therefore, the pedal triangle RST is also the medial triangle of triangle ABC, so its sides are parallel to each side of triangle ABC and are the half of their lengths.

This result implies another interesting relation. Since OS is perpendicular to AB, and AB is parallel to RT, we can easily conclude that if we extend OS to intersect RT, OS will be perpendicular to RT. The latter means that OS is altitude of pedal triangle RST. Similarly, if we extend OT to intersect RS and OR to intersect ST we will find that OT and OR are also altitudes of pedal triangle RST (by similar arguments that those already presented above). Thus, the Circumcenter of triangle ABC is also the Orthocenter of pedal triangle RST.

III. Pedal point as Orthocenter

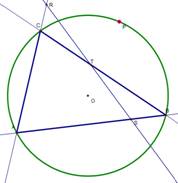
Given a triangle ABC, we can find its orthocenter and choose it as the pedal point for our pedal triangle RST. Figure 4 below shows the idea:

Figure 4

It is immediately followed from the definition of altitude and orthocenter that R, S and T, the vertices of the pedal triangle RST, are the foot of the altitudes of triangle ABC.

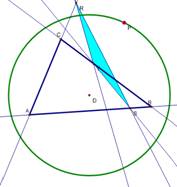
There is another relation which is more interesting. The Orthocenter of triangle ABC is the Incenter of pedal triangle RST. To prove this statement, we should consider figure 5 below:

Figure 5

First, it could be adequate to remember what a Cyclic Quadrilateral is. A cyclic quadrilateral is a quadrilateral that can be circumscribed by a circle. The cyclic quadrilaterals have the property that their opposite angles are supplementary. Also it could be useful to remember that any right angle can be circumscribed in a semi-circumference.

So, quadrilateral ASHR is cyclic since their opposite angles are supplementary, then quadrilateral ASHR could be circumscribed by the circle with center V (figure 5 above). At the same time, we can circumscribe triangles ASC and CTA by the same circle since both are right triangles and share the side AC, so quadrilateral ASTC is cyclic too (the circumcircle is circle with center U).

By the inscribed angle theorem, we can conclude that

Since , it follows that . Therefore, the altitude CS is also the angle bisector of angle RST. We could elaborate similar reasoning to prove that altitudes AT and BR are also the angle bisectors of angles RTS and SRT, respectively. Thus, we have shown that the orthocenter of triangle ABC is also the incenter of pedal triangle RST as shown in figure 6.

Figure 6

Thus, we have found an interesting “cyclic” relation between the triangle ABC’s centers and pedal triangle RST’s centers. Of course, we have to consider the adequate election of the pedal point to obtain one of the relations above shown. These relations are summarized as follow:

SUMMARY
Election of P (pedal point)	Relation
As incenter of DABC	The incenter of DABC is the circumcenter of the pedal triangle.
As circumcenter of DABC	The circumcenter of DABC is the orthocenter of the pedal triangle.
As orthocenter of DABC	The orthocenter of DABC is the incenter of the pedal triangle.

THE DEGENERATE CASE: THE SIMSON LINE

The election of P could involve that the points R, S and T were collinear. There is a limit case where those three points lie on the same line which is common called as Simson Line. Let’s consider the following examples:

Figure 7(a)	Figure 7(b)	Figure 7(c)

The sequence above suggests that the degenerate case appears when the pedal point is chosen such that it is on the circumcircle of triangle ABC. Let’s prove this statement.

Consider figure 8 below:

Figure 8

Let the green circle be the circumcircle of triangle ABC with circumcenter O, and let P be the pedal point on the circumcircle. Since PS is perpendicular to AS and PR is perpendicular to AR the quadrilateral ASPR is cyclic. A similar argument works to show that PRCT is a cyclic quadrilateral too.

Moreover, triangles PSB and PTB are right triangles and share the side PB, so they are circumscribed by the same circle. Therefore, quadrilateral PTSB is cyclic too.

We can also see that angle CAS is opposite the angles at P for both cyclic quadrilaterals ARPS and ACPB, then

Subtracting from both sides above, we will have

On the other hand, since quadrilateral PTSB is cyclic, we deduce

Also, we can note that quadrilateral RPTC is cyclic, so

Finally, by combining these latter results, we will have

Thus, points R, S and T are collinear and form the Simon Line.

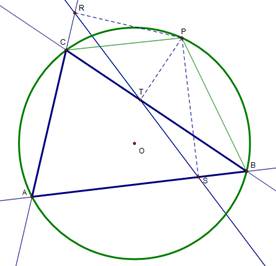
The Simson Line has an interesting characteristic which appears when we draw the trace of the line while the point P moves around the circumcircle. Figure 9 below shows the trace of Simson Line, but you can see an animation in GSP if you click here.

Figure 9

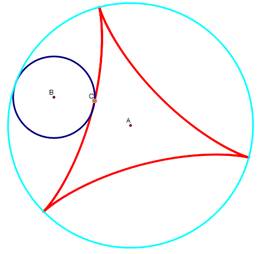
The curve drawn by the trace of Simson Line is known as Deltoid. The Deltoid could be defined the locus of a point on the circumference of a circle as it rolls without slipping along the inside of a circle with three times its radius. Figure 10 below shows the geometric definition of a Deltoid, but you can also see an animation if you click here.

Figure 10

We can observe that point C on the blue circle generates the Deltoid (red curve). The light-blue circle has a radius three times bigger as the radius of blue circle.